한정적분에 대한 심슨의 1/3 법칙

사다리꼴 규칙과 마찬가지로 Simpson의 1/3 규칙은 a에서 b까지의 범위에서 정수 값을 찾는 데에도 사용됩니다. 사다리꼴과 심슨의 1/3 법칙의 가장 큰 차이점은 사다리꼴 법칙에서는 전체 단면이 일부 사다리꼴로 분할되지만 이 경우 각 사다리꼴도 두 부분으로 분할된다는 점입니다.

이 규칙의 경우 다음 공식을 따릅니다.

여기서 h는 간격의 너비이고 n은 간격의 수입니다.

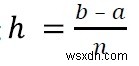
입력 및 출력

Input:
The function f(x): (x+(1/x). The lower and upper limit: 1, 2. The number of intervals: 20.
Output:
The answer is: 2.19315

알고리즘

integrateSimpson(a, b, n)

입력 - 적분의 하한 및 상한 및 간격 n.

출력 - 통합 후 결과입니다.

Begin
   h := (b - a)/n
   res := f(a) + f(b)
   lim := n/2

   for i := 1 to lim, do
      oddSum := oddSum + f(a + (2i - 1)h)
   done

   oddSum := oddSum * 4
   for i := 1 to lim-1, do
      evenSum := evenSum + f(a + 2ih)
   done

   evenSum := evenSum * 2
   res := res + oddSum + evenSum
   res := res * (h/3)
   return res
End

예시

#include<iostream>
#include<cmath>
using namespace std;

float mathFunc(float x) {
   return (x+(1/x));    //function 1 + 1/x
}

float integrate(float a, float b, int n) {
   float h, res = 0.0, oddSum = 0.0, evenSum = 0.0, lim;
   int i;
   h = (b-a)/n;    //calculate the distance between two interval
   res = (mathFunc(a)+mathFunc(b));    //initial sum using f(a) and f(b)
   lim = n/2;

   for(i = 1; i<=lim; i++)
      oddSum += mathFunc(a+(2*i-1)*h);    //sum of numbers, placed at odd number
   oddSum *= 4;    //odd sum are multiplied by 4

   for(i = 1; i<lim; i++)
      evenSum += mathFunc(a+(2*i)*h);    //sum of numbers, placed at even number
   evenSum *= 2;    //even sum are multiplied by 2
   res += oddSum+evenSum;
   res *= (h/3);
   return res;    //The result of integration
}

main() {
   float result, lowLim, upLim;
   int interval;
   cout << "Enter Lower Limit, Upper Limit and interval: ";
   cin >>lowLim >>upLim >>interval;
   result = integrate(lowLim, upLim, interval);
   cout << "The answer is: " << result;
}

출력

Enter Lower Limit, Upper Limit and interval: 1 2 20
The answer is: 2.19315